
There are many situations in both everyday life and sport activities in which bodies move with constant acceleration. Such motion is called uniformly accelerated motion. The condition here is that the resulting external force acting on the body is constant and non-zero.
Projectile vertical motion
“Projectile” is a term used in sport and physical exercise for any body that is launched, thrown, or shot into air in any direction, or simply dropped to the ground. A number of objects in sport and physical exercise can be considered to be projectiles: javelin, shot, discus, but also human body itself. Two forces always act on such projectiles:
- gravitational force
- resistance of the environment.
Sometimes the resistance is very small and can be neglected. In such cases only gravitational force acts on a projectile. In the previous chapter we already mentioned that gravitational acceleration g equals roughly 9,81 m/s2 and is oriented downward.
When neglecting friction (such as air resistance), projectiles always move in vertical direction either in uniformly decelerated motion (if going up) or uniformly accelerated motion (if falling down) with the gravitational acceleration of 9,81 m/s2.
Projectile motion can be described with equations that relate velocity, displacement, acceleration, and time. The following equation allows us to calculate the instanteous vertical velocity of a projectile at the end of any time interval Δt, given its initial velocity and the length of that time interval.
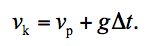
We can see that the vertical velocity of the projectile is in direct proportion to the time the projectile spent in the air. And do we know anything about the projectile’s position? The final position can be calculated as:

This equation allows us to calculate the vertical position of the projectile yk at the end of the time interval Δt, given its initial velocity vp and the length of that time interval.
Let us now analyze the motion of a ball dropped to the ground. Our equation will be markedly simpler as the initial velocity of the ball vp equals zero:

Vertical velocity of the free falling ball will then simply be:

Any object falling to the ground accelerates each second by 9,81 m/s2 and its distance travelled grows with the time squared.
Let us imagine a tennis service. To hit the ball correctly we need enough time for a proper stroke. Which position of the ball is the best for the stroke? When the ball is still going up, already going down, or when it stands still in the so called cuspidal point? We know that after tossing the ball up it starts to decelerate due to gravitation. It is easier to hit the ball if it moves slowly. Around the cuspidal point the ball moves very slowly, in the cuspidal point it even stops stops for an instant21 because its motion changes direction from upward to downward. The ball spends the longest time around the cuspidal point which makes the stroke easier.
Projectiles tossed vertically up have zero velocity in the cuspidal (highest) point of their trajectory and they spend the longest time around that point.
If tossing the ball in tennis service is considered to be vertical, interesting facts follow:
For vertical motion of a body in the Earth's gravitational field the following holds true:
- the time of ascending equals the time of descending,
- the initial velocity equals the impact velocity.
Projectile horizontal motion
Motions of bodies are mostly composed of vertical and horizontal components. For biomechanical analysis, however, it is useful to describe horizontal motion separately from vertical motion.
Horizontal velocity of projectiles is constant22 and the trajectory of horizontal motion is straight line.
We have derived equations for vertical motion and we can do the same for horizontal motion. Let us assume that the “x” axis of the system of Cartesian coordinates is horizontal and the motion of the projectile in question has positive direction on that axis. Then the following holds true:

Unless the horizontal velocity changes, the horizontal acceleration equals zero:

As horizontal velocity is constant, both mean and instanteous horizontal velocities throughout the whole time interval are identical. For the position of the projectile xk at the end of its motion the following holds true:

where xp and xk, respectively, stand for horizontal position at the beginning and at the end of the motion, respectively. If xp = 0, the equation for horizontal path can be simplified to:

Now we know all equations describing both vertical and horizontal motions of a projectile.
Angular Projection
Vertical and horizontal motions of a projectile are independent of each other, although we observe them at the same time. Let us express the time of horizontal motion:

and substitute it in the equation  which gives us
which gives us

Now we have the projectile position in both vertical and horizontal directions. However, we must know the initial vertical and horizontal components of velocity and the initial projectile position.
Generally the following holds true:
 23
23
which is the general equation of the parabola lying on the plane of axes “x” and “y”. Part of this parabola represents a set of positions through which the projectile moved during its motion.
Figure 9 Angular projection, assuming there is no air resistance.

Projectiles in Sport
Human body becomes a periodic projectile when running. Typical projectiles are: shot after being put, javelin after being thrown, football after being kicked, and human body in long jump, high jump, swimming (during starting dive), football (upward leap in preparation for a header), etc. In these situations, only gravitation acts on a projectile24. Even a human body, once it loses contact with the ground, cannot influence the trajectory of its flight which is fully controlled by the above equations25.
For the sporting purposes it can be said that the initial conditions (initial position and velocity) influence the following motion of the projectile and thus also the success of the given sporting action. In sporting actions such as throwing, putting, kicking, jumping, etc. the most important factors are:
- duration of flight
- maximum height reached by the projectile
- horizontal displacement.
Flight duration of projectiles in sport depends on the initial vertical velocity and initial vertical position. Falling object will fall longer from a higher initial position. If at the beginning of a fall a projectile already has certain initial velocity directed downwards, it will fall for a shorter period of time than in other cases. If a projectile is tossed upwards, the higher its initial velocity is, the longer it will stay airborne before falling back to the ground. All acrobatic jumps are a good example of maximisation of the flight duration in sport, when acrobats need enough time to perform all planned elements. In these sporting events the angle between the vector of initial velocity and the horizontal line (so called elevation angle) is close to 90°.
The maximum height reached by a projectile in sport depends mostly on the initial vertical velocity and position. A projectile tossed from a bigger height and with higher velocity will fly higher. Maximisation of the height reached by a projectile is important in sport events like volleyball and basketball, where athletes need to jump as high as possible. High jump is another example of maximisation of the height reached. In these activities the elevation angle is bigger than 45°.
Maximisation of horizontal displacement is the goal in many sporting events, for example: shot put, hammer throw, javelin, discus, long jump, etc. In discus and javelin the resistance of the environment is very important, therefore the following analysis will not apply to them. In other events mentioned the resistance of the environment is so weak it can be neglected and the basic knowledge following from our analysis can be applied here.
Let us start with the equation xk = vxΔt, which describes horizontal displacement as the function of horizontal velocity and flight duration. The bigger the horizontal velocity is, the further the projectile flies. We also know that the flight duration is longer with higher vertical velocity and position. This means that horizontal displacement will depend on three parameters at the moment of throwing (take-off):
- vertical velocity
- horizontal velocity
- vertical position.
In many cases the initial position is zero26, as is the case in many take-offs. Horizontal displacement depends only on vertical and horizontal velocities. Unless there are other forces acting on the projectile, it holds true that both horizontal and vertical velocities should have the same – and the highest possible – magnitude and the angle of take-off (throw) should be 45°. Let us have a look at the angle of throwing shot put. The best shot-putters in the world manage the angle of throwing of about 35°. That is much less than 45°. But what is the height of the throw? It is about 2 metres, depending on the height of the athlete. We know that the height at which the throw takes place influences the horizontal displacement.
The height of the throw gives the shot more time in the air, therefore it is better to increase horizontal velocity at the expense of vertical velocity.
Higher position of the projectile above the ground at the moment of the throw means lower need of vertical velocity for reaching maximum horizontal displacement – assuming the projectile will land on the ground.
McGinnis (2005) states another important reason why shot-putters produce higher horizontal component of velocity and therefore the angle of throwing smaller than 45°. Human body has developed in such a way that the arms can reach horizontal velocity against external resistance more easily than vertical velocity. This means that the resulting velocity of a throw decreases with the growing angle of the throw.
When we look at the angles of throwing javelin or discus we find out they are even smaller than the angle of throwing shot by the top shot-putters. During the flight of a shot, discus, or javelin the resistance of the environment generates lifting forces that cause the resultant acceleration to be lower than the gravitational acceleration. Therefore the discus or javelin stays in the air longer. For this reason the vertical velocity of the throw does not have to be very high. We should be reminded again that it is easier for an athlete to give horizontal velocity to a projectile, compared to vertical velocity. This is one of the reasons why the angle of the throw is relatively small. Frisbee throwing is an extreme example of making use of lifting forces. Here the lifting forces are so intense that the angle of the throw is almost zero.
21 Only if the ball is tossed up in vertical direction. If not, it stops moving in vertical direction for an instant.Zpět
22 If air resistance is neglected.Zpět
23 Holds true only if x = 0 at the beginning of motion.Zpět
24 Assuming there is no air resistance.Zpět
25 Assuming there is no resistance of the environment.Zpět
26 The position of the throw, as well as the position of the projectile at landing, have zero value on axis y.Zpět